Encyclopedia of Crystallographic Prototypes

Enantiomorphic Space Groups

Enantiomorphic space groups

In affine space — i.e., no defined origin — there are only 219 space groups (referred to as the affine space groups). The eleven remaining space groups are mirror images (left‐handed versus right‐handed structures) of one of the other 219 space groups and are equivalent in the affine space. These pairs of space groups are the enantiomorphic pairs, in which two prototypes can be formed as mirror images of a single structure. The eleven pairs of enantiomorphic space groups (Online Dictionary of Crystallography, ITC-A) are:

  • $P4_{1}$ (#76) and $P4_{3}$ (#78),
  • $P4_{1}22$ (#91) and $P4_{3}22$ (#95),
  • $P4_{1}2_{1}2$ (#92) and $P4_{3}2_{1}2$ (#96),
  • $P3_{1}$ (#144) and $P3_{2}$ (#145),
  • $P3_{1}12$ (#151) and $P3_{2}12$ (#153),
  • $P3_{1}21$ (#152) and $P3_{2}21$ (#154),
  • $P6_{1}$ (#169) and $P6_{5}$ (#170),
  • $P6_{2}$ (#171) and $P6_{4}$ (#172),
  • $P6_{1}22$ (#178) and $P6_{5}22$ (#179),
  • $P6_{2}22$ (#180) and $P6_{4}22$ (#181), and
  • $P4_{1}32$ (#213) and $P4_{3}32$ (#212).

The relationship between the enantiomorphic pairs is exploited in this encyclopedia to generate prototypes for otherwise unrepresented space groups. If we look at space group $P4_{1}$ (#76), we see that it has one Wyckoff position ($4a$), with operations (Bilbao Crystallographic Server):

\[ \left(x, y, z\right) \left(-x, -y, z + \frac{1}{2}\right) \left(-y, x, z + \frac{1}{4}\right) \left(y, -x, z + \frac{3}{4}\right). \]

If we then look at space group $P4_{3}$ (#78), we find it also has one ($4a$) Wyckoff position, with operations

\[ \left(x, y, z\right) \left(-x, -y, z + \frac{1}{2}\right) \left(-y, x, z + \frac{3}{4}\right) \left(y, -x, z + \frac{1}{4}\right), \]

where the only difference is that the 1/4 and 3/4 fractions have swapped positions. We can easily show that space group #78 is a mirror reflection of #76 in the $z = 0$ plane. To see this more clearly, consider the Cs3P7 structure (A3B7_tP40_76_3a_7a). This structure was found in space group #76, but if we reflect all of the coordinates through the $z = 0$ plane, it transforms into a structure in space group #78, as shown below. 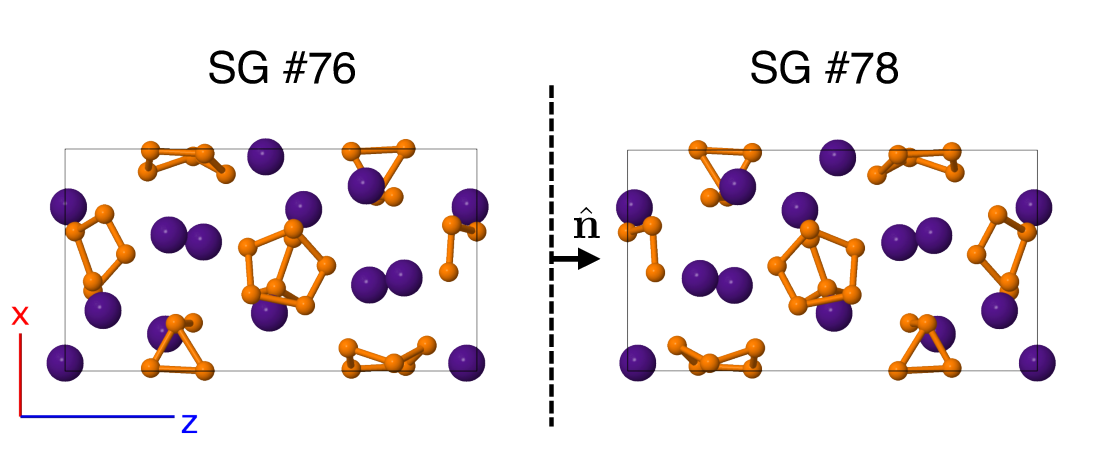./PICS/enantiomorph_example icon The distance between any pair of atoms is the same in the $P4_{3}$ structure as it is in the $P4_{1}$ structure, and the angle between any three atoms is the same in both structures. It follows that the structures are degenerate, there is no difference in energy between them, and they should be equally likely to form. Any structure in space group $P4_{1}$ can be transformed into $P4_{3}$ by this method. Pairs of space groups which allow these transformations are said to be enantiomorphic (Online Dictionary of Crystallography, ITC-A), or chiral. In addition, forty-three other space groups allow chiral crystal structures. The complete set of sixty-five space groups are known as the Sohncke groups (Online Dictionary of Crystallography).