The Triclinic Crystal System
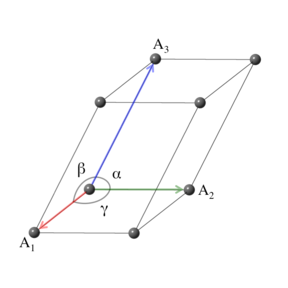
Triclinic is the most general crystal system. All other crystal systems can be considered special cases of the triclinic. The primitive vectors are also completely general: their lengths ($a$, $b$, $c$) and angles ($\alpha$, $\beta$, $\gamma$) may have arbitrary values, so long as the lengths are positive and the vectors do not form a plane ($\alpha = \beta = \gamma = 120^\circ$). The triclinic system has one Bravais lattice, which is also the conventional lattice for this system.
Lattice 1: Triclinic
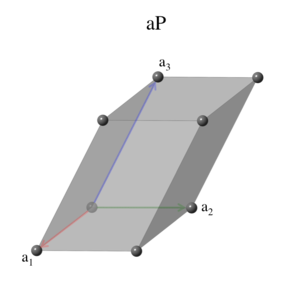
There are many choices for the primitive vectors in the triclinic system. We make the choice
\[ \begin{array}{ccc} \mathbf{a}_1 & = & a \, \mathbf{\hat{x}} \nonumber \\ \mathbf{a}_2 & = & b \, \cos\gamma \, \mathbf{\hat{x}} + b \, \sin\gamma \,\mathbf{\hat{y}} \nonumber \\ \mathbf{a}_3 & = & c_x \, \mathbf{\hat{x}} + c_y \, \mathbf{\hat{y}} + c_z \, \mathbf{\hat{z}}, \end{array} \]
where
\[ \begin{array}{ccc} c_x & = & c \, \cos\beta \\ c_y & = & \frac{c \, (\cos\alpha - \cos\beta \, \cos\gamma)}{\sin\gamma} \end{array} \] and \[ \begin{array}{ccc} c_z & = & \sqrt{c^2 - c_x^2 - c_y^2}. \end{array} \]
The volume of the triclinic unit cell is \[ V = a \, b \, c_z \, \sin\gamma. \] The space groups associated with the triclinic lattice are:
\[ \begin{array}{ll} 1. ~ \text{P1} & 2. ~ \text{P}\overline{1} \\ \end{array} \]